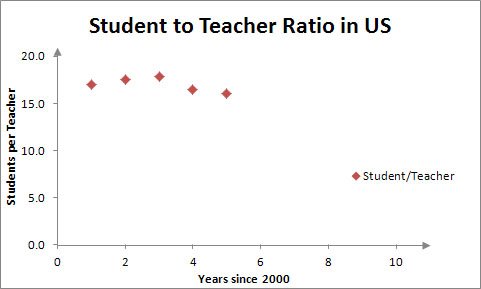
Most students begin graphing the student to teacher ratio in each year. They must calculate these ratios from the NCES data. As an example, the scatter plot of the student to teacher ratio for the entire United States from 2000 to 2006 looks like the graph below.
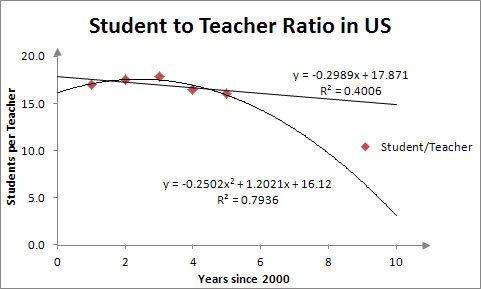
A superficial approach to this question is to model the student to teacher ratio with a linear or quadratic function. In the case of the entire US, this leads to functions that are decreasing.
Both of these functions predict that the student to teacher ratio will eventually reach zero. This is not likely to happen. What is more likely is that the ratio will begin to level off. To get that type of behavior, the students turn to modeling the students and teachers separately. The resulting functions are divided to give a ratio function.
Finally, students take the limit as the input approaches infinity to find the level the student to teacher ratio approaches in the long term.
As outlined with the US data, the strategy does not seem difficult to follow. However, each student is only using these graphs as a guide to complete a similar analysis on the state they have been assigned to analyze. Their data may or may not behave the same way as the US data. For instance, in many states the number of teachers is decreasing. Modeling the data with a polynomial leads to a function that will equal zero. The resulting ratio function then has a vertical asymptote. Students must model teachers with a function that is non-negative to eliminate this type of behavior. Most students examine several different options for their ratio to find one that behaves as they expect it should behave based on the data.