In the College Algebra course taught at Yavapai College, modeling data with different types of functions is a key focus. So the projects I use in my class utilize the same data and question. As noted in Part 1 of this post, the goal of this series of project is easy to understand.
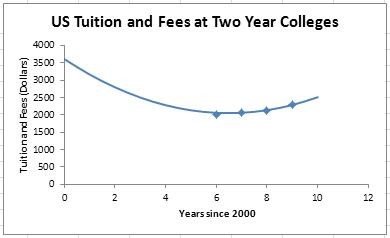
If you spend your first two years of college in a two-year college instead of a four-year college, how much would you save?
In the first project based on this question, a linear model is found for the data in the project. In subsequent projects, nonlinear models are calculated from the data.
The second project letter lays out the task for finding a quadratic model for the data.
College Cost (Quadratic) Project Letter (PDF)
This model is calculated through two technology assignments.
Technology Assignment – Use Matrices to Find a Model (PDF)
In this assignment, students duplicated the linear model they found earlier. This is done by substituting the two data values on the line into y = mt + b. Using the points (7, 2061) and (9, 2285), we get the system of equations
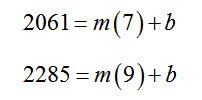
This corresponds to the augmented matrix
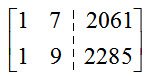
and reduced row echelon form
This means the linear model that passes through the two points is
y = 112t + 1277
This matches the model found in the first project by calculating the slope and vertical intercept. In the next technology assignment, this technique is applied to three data points and a quadratic model.
Technology Assignment – Find a Quadratic Function for College Costs (PDF)
If we use the points (7, 2061), (8, 2136) and (9, 2285) with the quadratic model y = at2 + bt + c, we get the system
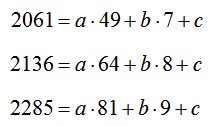
The augmented matrix for this system is

and the equivalent reduced row echelon form is

This gives the quadratic model
y = 37t2 -480t+3608
A graph of this function indicates that the model passes through the points correctly.

With the quadratic models for two-year and four-year colleges, students can find the savings from attending two year college for the first two years of obtaining a four-year degree.
This project puts quadratic functions in the context of matrices…two important parts of College Algebra at Yavapai College. A matrix approach like this would also be appropriate in Finite Mathematics.