The first two projects in the College Costs series focus on linear and quadratic models. In this post, we’ll look at exponential models of the college cost data and use it to answer the question: If you spend your first two years of college in a two-year college instead of a four-year college, how much would you save? We will demonstrate the process by modeling the national costs y as a function of the number of years after 2000 t with
y = Pert
This allows us to incorporate exponential function into the College Algebra curriculum as well as logarithms.
The project letter for this project is very similar to earlier project letters in the series.
College Costs (Exponential) Project Letter (PDF)
The goal of this project is to find the savings using exponential models for two-year and four-year college costs. These models will pass through two of the data points for each dataset. Students complete one technology assignment to help them find the model.
Technology Assignment – Find an Exponential Function for College Costs (PDF)
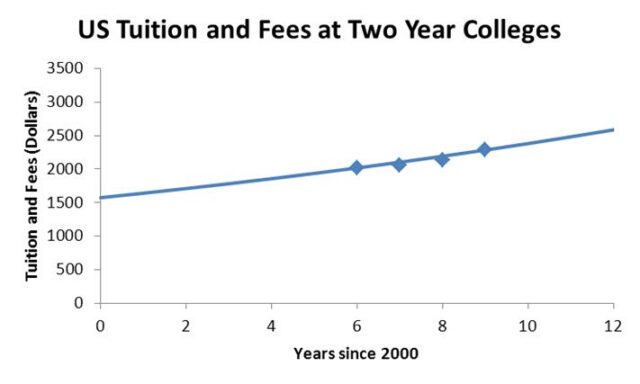
For the US two-year college data at (6, 2018) and (9, 2285), we substitute the values into the model to obtain the nonlinear system,
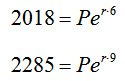
If we can solve this system of equations for P and r, we’ll have the constants in the exponential model. Solve the first equation for P to give
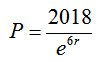
This can be substituted into the second equation in the system to yield,
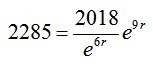
Solving for r gives
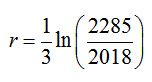
Using these values in the model gives the graph shown below.
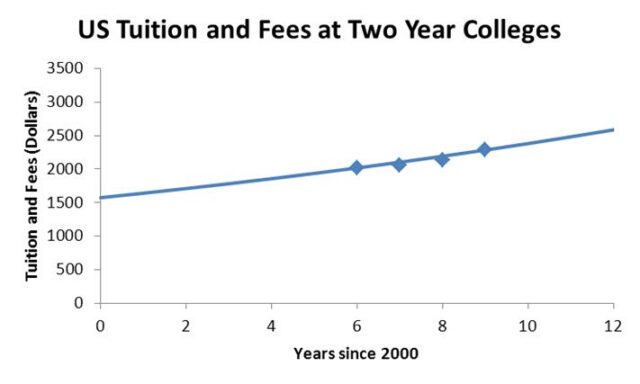
Denote national two-year college costs as T(t) and four-year college costs as F(t) where t is the number of years after 2000. As in earlier projects, the savings are
Savings = F(13) + F(14) – T(13) – T(14)
over the period 2013-2017.
The fourth project functions as a final in my College Algebra class. As with the earlier project, it focuses on the savings accrued from attending two-year college to earn a four-year degree. In this project, students find a logarithmic regression model of the form
y = a + b ln(x)
Since this takes the place of a final exam, each student is on their own to find the model. No technology assignments are assigned. Using some type of technology, students produce a logarithmic function like the one below and use it to calculate the savings.
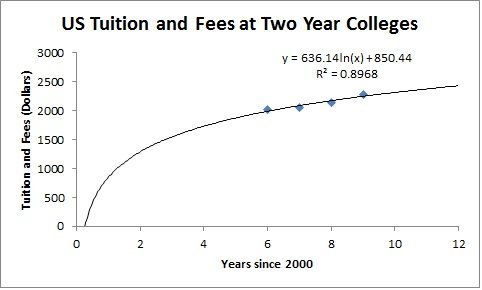
This series of projects exposes students to thee key concepts in college algebra repeatedly. This repeated retrieval helps them to learn exactly what a model is and how it might be used. The projects are far from easy and have many possible tangents that students may focus on. This effort forces them to really understand what they are doing.












